The propagation of quantum information within interacting boson systems has long been a topic of interest for scientists studying quantum many-body systems. These systems, which include bosons like those found in Bose-Einstein condensates (BECs), play a crucial role in various branches of physics. Dr. Tomotaka Kuwahara and his team from Japan’s RIKEN Center for Quantum Computing recently conducted a study to explore the transmission of quantum information within interacting boson systems. Their findings challenge the conventional wisdom about the speed at which information can travel in these systems.
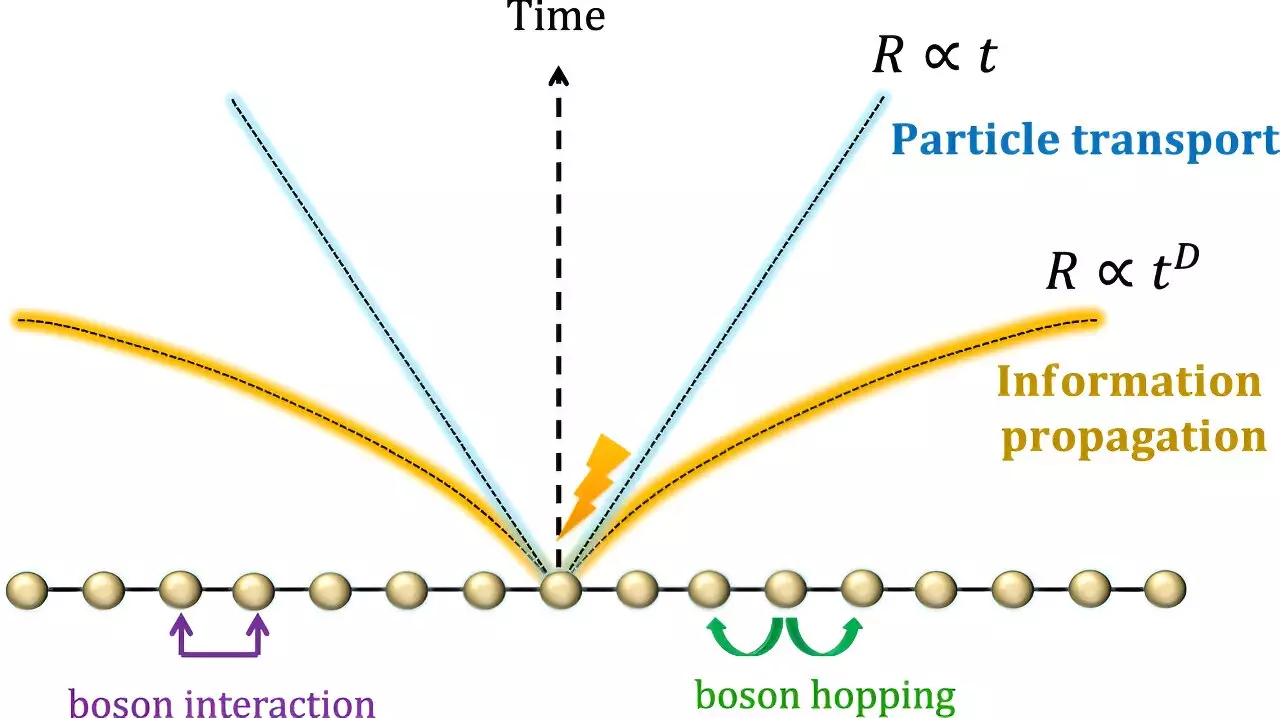
The Lieb-Robinson bound is a fundamental concept in quantum mechanics that quantifies the speed at which information or changes can propagate through a quantum system. In the context of interacting boson systems, the Lieb-Robinson bound has presented a significant challenge due to the unbounded energy and long-range interactions inherent in these systems. However, Dr. Kuwahara and his team sought to address this challenge by studying the propagation of quantum information in a D-dimensional lattice governed by the Bose-Hubbard model.
The researchers made several key discoveries in their study of interacting boson systems. One of the most significant findings was that the speed of boson transport is limited in these systems, even with long-range interactions. This speed was found to grow at most logarithmically with time, indicating that information propagation is relatively slow in interacting boson systems. Additionally, the researchers found that interactions among bosons induce clustering in specific regions, facilitating accelerated information propagation along certain lattice paths. This phenomenon, while bounded, provides valuable insights into the dynamics of boson systems.
One interesting comparison highlighted in the study is the difference in information propagation speeds between bosonic and fermionic systems. While fermionic systems exhibit a finite speed limit for information propagation, bosonic systems show a different behavior. Dr. Kuwahara explained that bosons can send information much faster than fermions, especially as more bosons cooperate over time. This non-linear acceleration of information propagation in bosonic systems opens up new possibilities for studying quantum thermalization and simulating condensed matter physics.
The findings of this study have important implications for the field of quantum computing. By understanding the limits and dynamics of information propagation in interacting boson systems, researchers can develop more efficient simulations and theoretical models for studying quantum many-body systems. The use of elementary quantum gates, such as CNOT gates, to simulate the time evolution of interacting boson systems represents a promising avenue for further exploration in the field of quantum computing. Dr. Kuwahara anticipates that these findings will lead to the discovery of new quantum phases and aid in simulating quantum thermalization processes in closed quantum systems.
The study by Dr. Kuwahara and his team sheds new light on the accelerated transmission of quantum information in interacting boson systems. By challenging existing notions about the speed limits of information propagation in these systems, the researchers have opened up new possibilities for exploring the dynamics of quantum many-body systems. This work not only advances our understanding of fundamental particles like bosons but also paves the way for innovative applications in quantum computing and condensed matter physics.
Leave a Reply