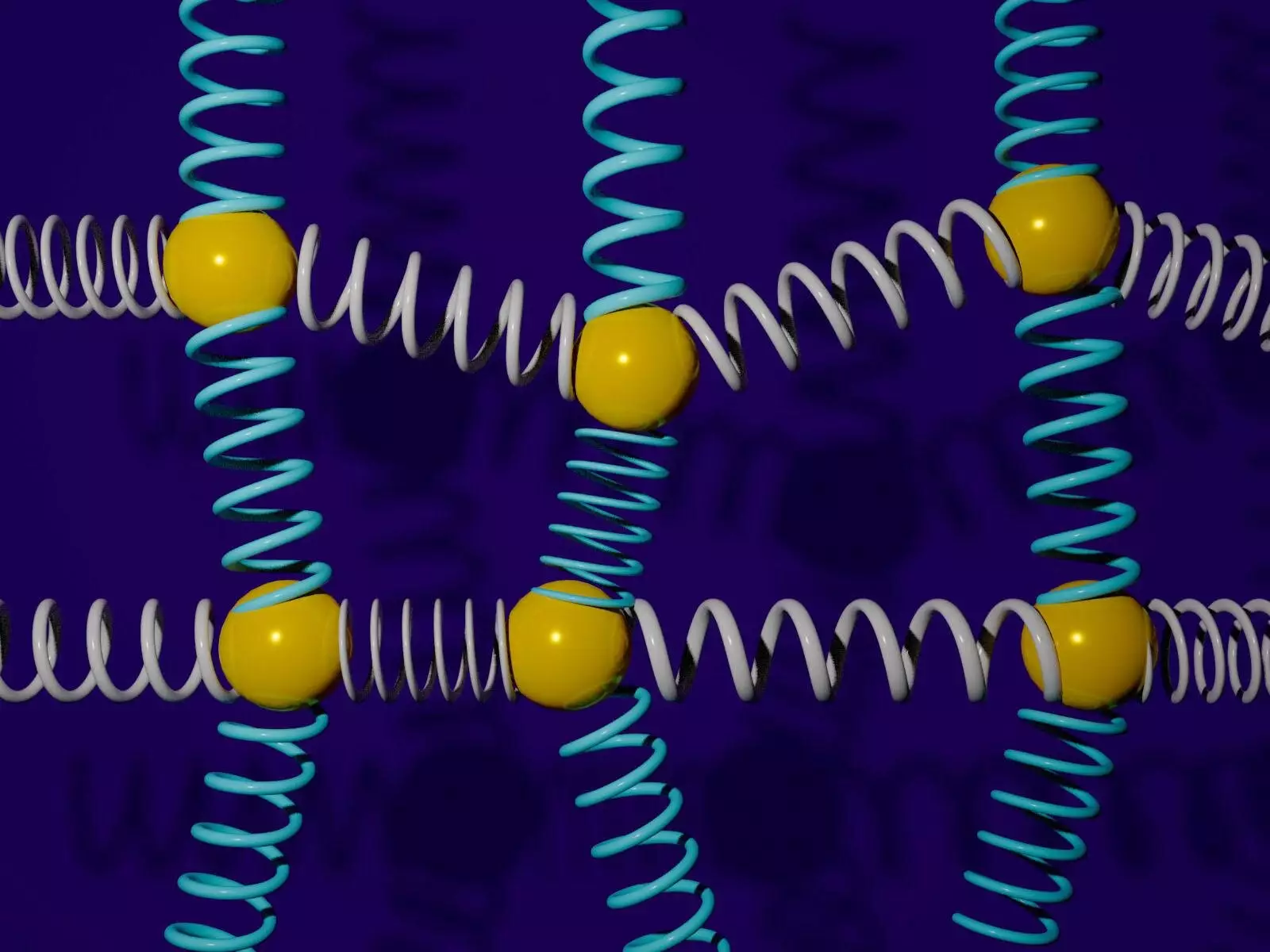
Coupled oscillations, while perhaps not a widely recognized term, play a significant role in various natural phenomena. From describing interacting systems of masses and springs to gravitational tidal effects between celestial bodies, the importance of coupled harmonic oscillators extends across different scientific disciplines. Recent developments in quantum computing have opened up new possibilities in simulating and understanding such complex systems, offering exponential speedups over classical algorithms.
A groundbreaking quantum algorithm, devised in part by researchers at Pacific Northwest National Laboratory and the University of Toronto, has revolutionized the simulation of coupled oscillator systems on quantum computers. By mapping the dynamics of these oscillators to a Schrödinger equation, researchers were able to simulate the system using Hamiltonian methods, significantly reducing the number of quantum bits required for the simulation. This innovative approach not only streamlines the simulation process but also highlights the potential of quantum computing in tackling complex problems.
The exponential advantage demonstrated by the new quantum algorithm raises intriguing questions about the future of computational science. By showcasing the ability of coupled harmonic oscillators to emulate quantum computers, the research suggests that large systems of interacting masses and springs could possess computational capabilities equivalent to quantum systems. This finding challenges conventional notions of computational power and sheds light on the intricate relationship between quantum dynamics and classical oscillatory systems.
The study’s exploration of theoretical constraints surrounding the calculation of these dynamics offers valuable insights into the nature of quantum computing. If classical computers could simulate these dynamics in polynomial time, it would imply that quantum computers are not fundamentally more powerful than their classical counterparts. However, existing evidence strongly indicates the superior computational capabilities of quantum systems, reinforcing the notion of exponential speedups offered by quantum algorithms. This research thus paves the way for further investigations into the intersection of quantum mechanics and classical oscillatory systems.
The development of a novel quantum algorithm for simulating coupled oscillatory systems marks a significant advancement in computational science. By leveraging the inherent efficiencies of quantum computing, researchers have unlocked new possibilities for studying complex phenomena across varied disciplines. The exponential speedup offered by this algorithm not only demonstrates the potential of quantum technologies but also underscores the intricate connections between quantum dynamics and classical harmonic oscillators. As we continue to delve deeper into the realm of quantum computing, such innovations hold the promise of reshaping our understanding of the natural world and pushing the boundaries of computational efficiency.
Leave a Reply